Central Tendency

Central Tendency

• There are 3 primary measures of central tendency; each has pros and cons, but all attempt to describe the center point of a distribution of scores.
  • Mode
  • Median
  • Mean
• Measures of central tendency offer us a ``point'' estimate, or single number, which we can use as a summary of a distribution of scores.
  • One number which represents or characterizes the entire distribution (as best as one number can).
• Keep in mind, the center point of scores in a distribution may not be in the middle of the scale of those scores (more on this later).

The Mode: symbol = Mo

The mode is the most frequently occurring score in a distribution.

• Commonly used with categorical variables.
• Pro: Easy to compute: simply observe and report the most frequent score(s).
• Pro: Not affected by outliers
• Con: Usually only reflects one actual score¹.
• Con: Different samples almost always produce different modes for the same variable.

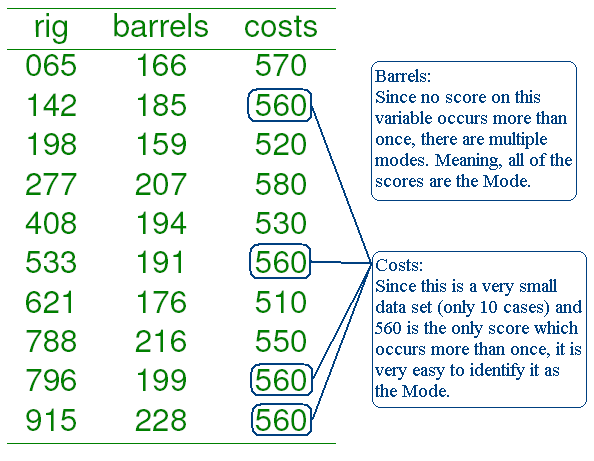
Oil Sample (n = 10) Example showing the Mode

The Median: symbol = Mdn

The median is the ``middle'' score of a distribution.

• More precisely, it is the point that lies in the middle of a distribution.
  • Sometimes referred to as the 50th percentile because, there are as many scores above it as there are below it.
• Pro: Not affected by outliers (extreme scores).
• Con: Ignores all but the middle of a distribution.

To calculate the median, first the scores must be arranged in sequential order (e.g., smallest to largest).

• Then;
  • For an odd number of scores, the middle score is the Median.
  • For an even number of scores, the average of the two middle scores is the median.

Oil Sample (n = 10) Example showing the Median

The Mean: sample symbol = 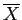, population symbol =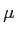

The mean is the arithmetic average of the scores of a distribution.

• Mean is the most popular measure of central tendency.
• Pro: Generally the best measure of central tendency because, it utilizes all the scores.
• Con: Very sensitive to outliers (extreme scores).

To calculate the sample mean, simply sum all of the scores and divide by the number of scores:

Oil Sample (n = 10) Example showing the Mean

General formula for Mean:

or²:

Barrels:

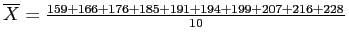
• Barrels:

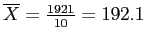
Costs:

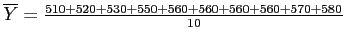
• Costs:
  

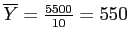
Trimmed Mean & M-estimators

Because mean is very sensitive to outliers, alternatives have been proposed which attempt to correct for this problem.

• Trimmed mean simply refers to a mean calculated after ``trimming'' a certain percentage of extreme scores.
  • The median is an extreme example of a trimmed mean; the median trims all but the middle score or middle two scores.
  • Common examples are 10% and 20% trimmed means; where the 10 or 20% of the most extreme scores (high & low) are trimmed.
• M-estimators are weighted means; meaning scores near the middle are given more weight and scores at the extremes are given less weight.